Fractional-Order Models for HIV Viral and Epidemiological Dynamics

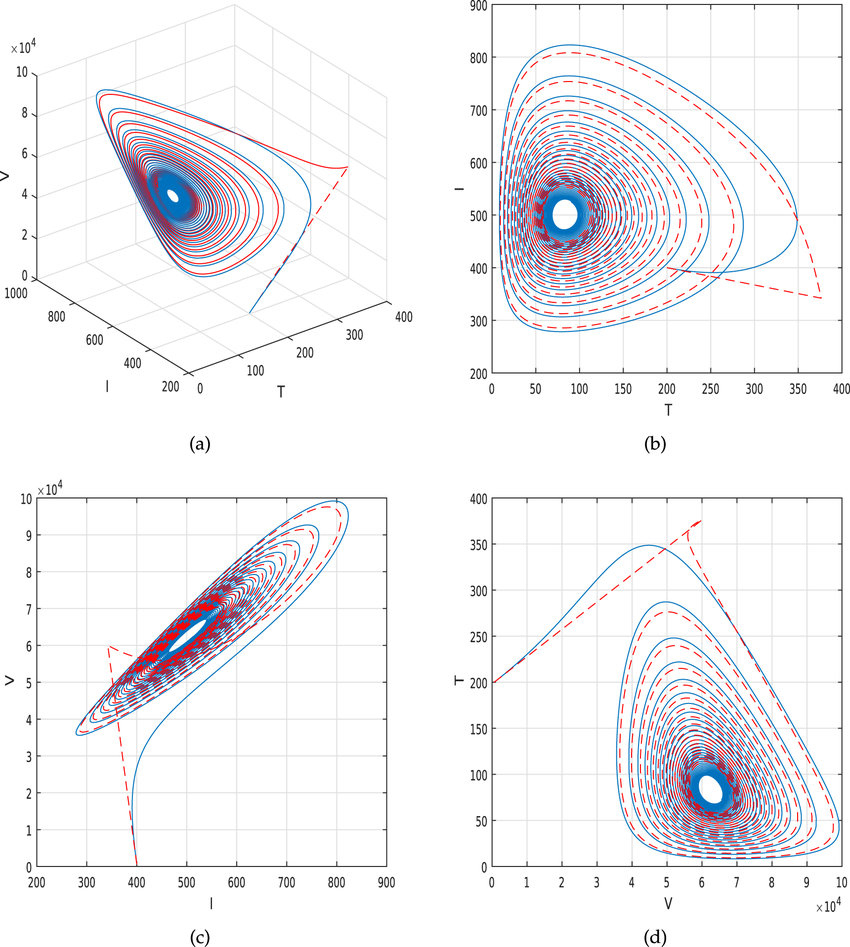
Since 1981, millions have died of HIV with no available cure until now. Therefore, researchers have always tried to study its dynamics through mathematical modeling to make it more predictable and controllable. In this chapter, the authors are concerned with two different models: the viral and epidemiological dynamics of HIV. Commonly, researchers introduce HIV dynamics using ordinary differential equations; however, here, the models are introduced as fractional-order systems that are considered more accurate and flexible. For the viral dynamics model, most of the studies focus on one target cell. Nevertheless, the authors here concentrate on two different cells: the CD4+ T cells and the macrophage cells. For the epidemiological dynamics model, a new term called the recovery rate is introduced. The two systems are simulated using the fractional predictor corrector method on MATLAB and the stability of both systems is verified. © 2018 Elsevier Inc. All rights reserved.